HBSE Class 10 Math Important Questions Answer 2025 PDF

Class 10 Mathematics Haryana Board Solution for Important Question Answer for HBSE. CCL Chapter Provide Class 1th to 12th all Subjects Solution With Notes, Question Answer, Summary and Important Questions. Class 10 Math mcq, Important Question Answer, Textual Question Answer are available of HBSE Board.

Also Read – Class 10 Math NCERT Solution
Also Read – Class 10 गणित NCERT Solution

HBSE Class 10 math Important Question with Answer for Haryana Board Solution.

HBSE Class 10 Math Important Question Answer 2025

हरियाणा बोर्ड 2025 की Paid PDF खरीदें और पेपर में करें 90% + स्कोर – वेबसाइट पर खरीदते समय कूपन कोड ‘CCL‘ इस्तेमाल करें और PDF की कीमत घटाएं

PDF को टेलीग्राम से खरीदने के लिए मैसेज करें – Hlo CCL (Telegram Link)

HBSE Class 10 Math Chapter 1 Real Number Important Questions 2025

Q1. Find HCF of 510 and 92. Most Most Important

Q2. Prove that $\displaystyle \sqrt{5}$ is an irrational number. Most Important

Q3. Prove that $\displaystyle \sqrt{3}$ is an irrational number. Most Important

Q4. Prove that $\displaystyle \sqrt{2}$ is an irrational number. Most Important

Q5. Prove that $\displaystyle 3\sqrt{2}$ is an irrational number. Most Important

Q6. Show that $\displaystyle 5-3\sqrt{2}$ is an irrational number.

Q7. Show that 7+ √5 is an irrational number.

Q8. $\displaystyle 3+2\sqrt{5}$ is a rational number or irrational number.

Q9. Express 3825 as a product of prime factors: Most Important

HBSE Class 10 Math Chapter 2 Polynomials Important Questions 2025

Q1. Find a quadratic polynomial, the sum and product of whose zeroes are -3 and 2 respectively. Most important

Q2. Find the product of zeroes of quadratic polynomial x² + 7x + 10. Most important

Q3. Find a quadratic polynomial with the given number as the sum and product of its zeroes respectively are 5 and 3. Most Important

Q4. Find a quadratic polynomial whose zeros are -3 and 5. Most Important

Q5. Product of roots of the quadratic polynomial 6x² – 7x – 3 is ____________.

Q6. Sum of the roots of the quadratic polynomnial 7x² – 3x + 1 is __________.

Q7. Find the sum of zeroes of quadratic polynomial x² – 2x – 8. Most important

HBSE Class 10 Math Chapter 3 Pair of Linear Equations in Two Variables Important Questions 2025

Q1. Solve the following pair of linear equations: 2x + 3y = 7 and 6x – 5y = 11.

Q2. For what value of k does the following pair of linear equations have infinite number of solutions? (k – 1)x + (k + 1)y = 3k – 1 and 2x + 3y = 7

Q3. The pair of linear equations 3x + 5y = 7 and 9x – 10y = 14 is consistent or inconsistent.

Q4. For what values of K does the pair of linear equations 4x + Ky + 8 = 0 and 2x + 2y + 2 = 0 has unique solution?

Q5. For what values of K does the pair of linear equations x – ky + 4 = 0 and 2x – 6y – 5 = 0 has no solution.

Q6. Solve: x + y = 5 and 2x – 3y = 4

Q7. If we add 1 to the numerator and subtract 1 from the denominator, a fraction reduces to 1. It becomes $\displaystyle \frac{1}{2}$ if we only add 1 to denominator. Find the fraction. Most Important

Q8. Five years hence, the age of Jacob will be three times that of his son. Five years ago, Jacob’s age was seven times that of his son. What are their present ages ?

Q9. Five years ago, Nuri was thrice as old as Sonu. Ten years later, Nuri will be twice as old as Sonu. How old are Nuri and Sonu ?.

Q10. The sum of the digits of a two-digit number is 9. Also nine times this number is twice the number obtained by reversing the order of the digits. Find the number.

HBSE Class 10 Math Chapter 4 Quadratic Equations Important Questions 2025

Q1. Find the root of the quadratic equation by factorization method $\displaystyle \sqrt{2}{{x}^{2}}+7x+5\sqrt{2}=0$

Q2. For what value of k the roots of the quadratic equation 2x² + kx + 3 = 0 are equal?

Q3. Sum of the roots of the quadratic polynomial 7x² – 3x + 1 is __________.

Q4. Factorise the quadratic equation 2x² + x – 6 = 0 into linear factors.

Q5. The roots of the quadratic equation 6x² – x – 2 = 0 are __________.

Q6. For what values of K, quadratic equation x² + Kx + 4 = 0 has equal roots?

Q7. Find discriminant of quadratic equation x² + 7x – 60 = 0.

Q8. Semi perimeter of a rectangular garden, whose length is 4m more: than its width is 36m. Find the dimensions of the garden.

Q9. Find the nature of roots of the following quadratic equation. If real root exist, then solve it: 2x² – 6x + 3 = 0

Q10. Find two consecutive positive integers the sum of whose squares is 365.

Q11. Find two numbers whose sum is 37 and product is 300.

Q12. Find two numbers whose sum is 27 and product is 182.

Q13. Is it possible to design a rectangular mango grove whose length is twice its breadth and area is 800 m²? If so, find its length and breadth.

Q14. Is the following situation possible? If so, determine their present ages. The sum of the ages of two friends is 20 years. Four years ago, the product of their ages in years was 48.

Q15. One side of a rectangle exceeds its other side by 2cm. If its area is 195 cm² , then determine the sides of the rectangle.

HBSE Class 10 Math Chapter 5 Arithmetic Progression Important Questions 2025

Q1. If the sum of first 7 terms of A. P. is 49 and sum of first 17 terms is 289, then find the sum of n terms of A. P. Most Important

Q2. If the sum of first 6 terms is 12 and sum of first 10 terms is 60, then find the sum of its n terms.

Q3. If the sum of first 10 terms of an A. P. is -60 and sum of first 15 terms is-165, then find the sum of its n terms.

Q4. The first term of an A.P. is 5, the last term is 45 and the sum is 400. Find the number of terms and common difference::

Q5. How many terms of A. P. : 9, 17, 25, ………. Should be taken so that their sum is 636 ?

Q6. Which term of A.P. 3, 15, 27, 39………… will be 132 more than its 54th term?

Q7. Find the 20th term from the last of the A. P. 3, 8, 13, …………, 253.

Q8. Find the 31st term of an A. P. whose 11th term is 38 and 16th term is 73.

Q9. The sum of first n natural numbers is __________.

Q10. The sum of first 50 natural numbers is ________ .

Q11. Write the next four terms of the A. P. 1, -1, -3, -5, ……………

Q12. Find the sum of first 10 terms of A.P. 2, 7, 12, 17, …………..

Q13. Find 11th term of A.P. 7, 13, 19 …………. Most Important

Q14. Find the common difference of the A.P. : 3, 1, −1, -3, ……………. Most Important

Q15. Find common difference of AP. 7, 5, 3, 1 ……………

Q16. How many three digit numbers are divisible by 7 ?

Q17. How many two-digit numbers are divisible by 3?

Q18. Find the sum of first 40 positive integers divisible by 6.

Q19. How many multiples of 4 lie between 10 and 250 ?

Q20. How many terms of sequence 1, 4, 7, 10, ………. should be taken so that their sum is 176 ?

HBSE Class 10 Math Chapter 6 Triangles Important Questions 2025

Q1. Two polygons of the same number of sides are similar, if their corresponding angles are ___________. (equal, proportional)

Q2. If areas of two similar triangles are equal, then prove that they are congruent.

Q3. All squares are ___________. (similar, congruent) Most Important

Q4. All __________ triangles are similar. (isosceles, equilateral) Most Important

Q5. Sides of triangles are given below. Determine which of them is a right triangle (i) 3 cm, 8 cm, 6 cm (ii) 13 cm, 12 cm, 5 cm

Q6. A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2 m/s. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.

Q7. In figure DE || BC. Find the value of EC: Most Most Important

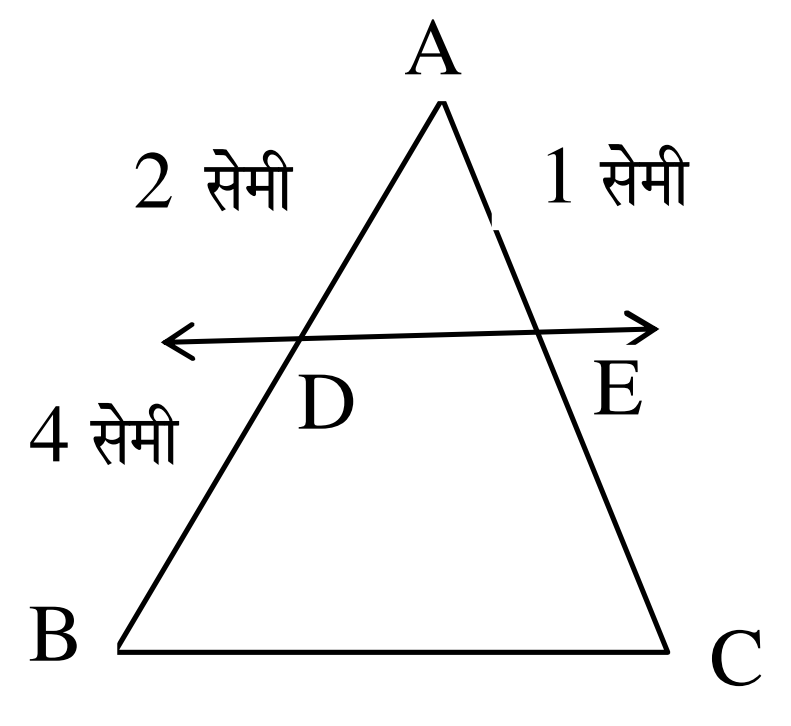
HBSE Class 10 Math Chapter 7 Coordinate Geometry Important Questions 2025

Q1. Find the distance between the points (-5, 7) and (-1, 3). Most Important

Q2. Find the ratio in which the line joining (3, 4) and (-4, 7) is divided by y- axis. Also find the coordinates of the point of intersection. Most Important

Q3. Find the distance between points (a, b) and (-a, -b).

Q4. If (3, 4) is mid point of the line segment whose one end is (7, -2), then find the coordinates of the other end point.

Q5. Find the mid point of the line segment whose end points are (4, 5) and (2, -1).

Q6. Find the mid point of the line joining the points (4, 7) and (2, 3).

Q7. If origin is at one end of a line segment whose mid point is (1, 0), find the coordinates of other end of segment.

Q8. Find the co-ordinates of a point A where AB is the diameter of a circle whose centre is (2, -3) and co-ordinates of B is (1, 4).

Q9. If the points A(6, 1), B(8, 2), C(9, 4) and D(p, 3) are the vertices of a parallelogram, taken in order, find the value of p.

Q10. Find the ratio in which the y-axis divides the line segment joining the points (5, 6) and (-1, -4). Also find the point of intersection.

Q11. Find the ratio in which the line segment joining the points (-3, 10) and (6, -8) is divided by (-1, 6).

Q12. Find the co-ordinates of the points of trisection of the line segment joining the points A(2, -2) and B(-7, 4).

Q13. In what ratio does the point (-4, 6) divides the line segment joining the points A(-6, 10) and B(3, -8).

Q14. Find the coordinates of the point which divide the line segment joining the points (4, -3) and (8, 5) in a ratio 3 : 1 internally. Most Important

HBSE Class 10 Math Chapter 8 Introduction to Trigonometry Important Questions 2025

Q1. Prove that : $\displaystyle \frac{{1+\sec A}}{{\sec A}}=\frac{{{{{\sin }}^{2}}A}}{{1-\cos A}}$

Q2. Prove that : $\displaystyle \frac{{\tan \theta }}{{1-\cot \theta }}+\frac{{\cot \theta }}{{1-\tan \theta }}$ = 1 + secθ cosecθ

Most Important

Q3. Prove that : (sin A + cosec A)² + (cos A + sec A)² = 7 + tan²A + cot²A

Q4. Prove that $\displaystyle \frac{{\cos A+\sin A-1}}{{\cos A-\sin A+1}}=\frac{1}{{\cos ecA+\cot A}}$ , using the identity cosec²A- cot²A = 1.

Q5. Prove that: $\displaystyle \frac{{\sin \theta +\cos \theta -1}}{{\sin \theta -\cos \theta +1}}=\frac{1}{{\sec \theta +\tan \theta }}$

Most Important

Q6. Prove that: $\displaystyle \frac{{\cos A-\sin A+1}}{{\cos A+\sin A-1}}=\cos ecA+\cot A$

Q7. Prove that : $\displaystyle \frac{{\cos \theta }}{{1+\sin \theta }}+\frac{{\cos \theta }}{{1-\sin \theta }}=2\sec \theta$

Q8. Prove that : $\displaystyle \sqrt{{\frac{{1+\sin A}}{{1-\sin A}}}}$ = sec A + tan A

Q9. Prove that: (cosec θ – cot θ)² = $\displaystyle \frac{{1-\cos \theta }}{{1+\cos \theta }}$

Q10. Prove that: $\displaystyle \frac{{\cos A}}{{1+\sin A}}+\frac{{1+\sin A}}{{\cos A}}$ = 2sec A

Q11. Prove that: (cosecA – sinA) (secA – cosA) (tanA + cotA) = 1

Q12. Prove that : $\displaystyle \frac{{\sin \theta -\cos \theta +1}}{{\sin \theta +\cos \theta -1}}=\frac{1}{{\sec \theta -\tan \theta }}$

Q13. Find the value of sin 45° + cos 45°. Most Important

Q14. If sin A = $\displaystyle \frac{4}{5}$ , find the value of cos A.

Q15. If tan (A + B) = $\displaystyle \sqrt{3}$ and tan(A – B) = $\displaystyle \frac{1}{{\sqrt{3}}}$ , 0° < A + B ≤ 90°, A > B, then find the value of A and B.

Most Important

Q16. If sin(A + B) = $\displaystyle \frac{{\sqrt{3}}}{2}$ and sin(A – B) = $\displaystyle \frac{1}{2}$ , 0° < A + B ≤ 90°, A > B, then find A and B.

Q17. The value of 1 + tan² θ = ___________.

Q18. Find the value of $\displaystyle \frac{{2\tan {{{45}}^{\circ }}}}{{1+{{{\tan }}^{2}}{{{45}}^{\circ }}}}$

Q19. Prove that : $\displaystyle \frac{{\cot A-\cos A}}{{\cot A+\cos A}}=\frac{{\cos ecA-1}}{{\cos ecA+1}}$

Q20. Prove that : sec A (1 – sin A) (sec A + tan A) = 1

Q21. In ΔOPQ, right-angled at P, OP = 7 cm and OQ – PQ = 1 cm. Determine the values of sin Q and cos Q.

Q22. If sin(A-B) = $\displaystyle \frac{1}{2}$ , cos(A+B)= $\displaystyle \frac{1}{2}$ , 0° < A + B ≤ 90°, A > B, find A and B.

Q23. In ΔPQR, right-angled at Q, PR + QR = 25 cm and PQ = 5 cm. Determine the values of sin P.

Q24. “cosθ = $\displaystyle \frac{3}{2}$ for some angle θ” (true / false)

Q25. “sinθ= cosθ for all values of θ” (true / false)

Q26. “The value of tanA is always lies in between -1 and 1” (true / false)

Q27. “cotA is not defined for A = 0° ” (true / false)

Q28. “The value of secA is always lies between -1 and 1” (true / false)

Q29. “cosec A is the product of cosec and A” (true / false)

Q30. “The value of cosecθ lies ≥ 1 and ≤ 1 ” (true / false)

Q31. “sinθ = 3/2 for some angle θ ” (true / false)

Q32. Evaluate: $\displaystyle \frac{{7{{{\sin }}^{2}}{{{30}}^{\circ }}+6\cos e{{c}^{2}}{{{60}}^{\circ }}-{{{\cot }}^{2}}{{{45}}^{\circ }}}}{{{{{\sin }}^{2}}{{{60}}^{\circ }}+{{{\cos }}^{2}}{{{60}}^{\circ }}}}$

Q33. Evaluate: $\displaystyle \frac{{5{{{\cos }}^{2}}{{{60}}^{\circ }}+4{{{\sec }}^{2}}{{{30}}^{\circ }}-{{{\tan }}^{2}}{{{45}}^{\circ }}}}{{{{{\sin }}^{2}}{{{30}}^{\circ }}+{{{\cos }}^{2}}{{{30}}^{\circ }}}}$

Q34. If sec θ = $\displaystyle \frac{{13}}{{12}}$ ,then find sin θ.

Q35. The value of 2tan² 45° + cos² 30° – sin² 60° is

Q36. sin 60° cos 30° + sin 30° cos 60° is equal to _________.

Q37. The value of cos² θ + sin² θ = __________.

Q38. If sin A = $\displaystyle \frac{3}{4}$ , find value of cos A.

Q39. If cos A = $\displaystyle \frac{{12}}{{13}}$ find the value of tan A.

HBSE Class 10 Math Chapter 9 Some Application of Trigonometry Important Questions 2025

Q1. The shadow of a tower standing on a level ground is found to be 40 m longer when the altitude (the angle of elevation) of sun changes from 60° to 30°. Find the height of tower.

Q2. The angle of elevation of the top of a building from foot of tower is 30° and angle of elevation of top of the tower from the foot of building is 60°. If height of tower is 50 m. Find the height of building.

Q3. From the top of a 7 m building the angle of elevation of the top of a tower is 60° and angle of depression is 45°. Find the height of the tower. Most Important

Q4. The angle of elevation of the top of a tower from a point on the ground, which is 20 m away from the foot of the tower, is 30°. Find the height of the tower.

Q5. An observer 1.6m tall is 20m away from a tower. The angle of elevation of the top of the tower from his eyes is 60°. Find the height of the tower? Most Important

Q6. A tower stands vertically on the ground. From a point on the ground, which is 15 m away from the foot of the tower, the angle of elevation of the top of the tower is found to be 60°. Find the height of the tower.

Q7. A wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that wire will be taut ?

Q8. A ladder is placed against a wall such that its foot is at a distance of 2.5m from the wall and its top reaches a window 6m above the ground. Find the length of the ladder.

Q9. A vertical pole of length 6m casts a shadow 4m long on the ground and at the same time a tower casts a shadow 28m long. Find the height of the tower.

Q10. A person goes 10 m due east and then 30 m due north. Find the distance from the starting point.

Q11. A ladder 17 m long reaches a window of a building 15 m above the ground, find the distance of the foot of the ladder from the building.

Q12. Two poles of heights 6 m and 12 m stand on a level plane ground. If the distance between the feet of the poles is 8 m, then find the distance between their tops.

Q13. From a point on the ground, the angles of elevation of the bottom and top of a transmission tower fixed at the top of a 20m high building are 45° and 60° respectively. Find the height of the tower.

Q14. From a point on a bridge across a river, the angles of depression of the banks on opposite sides of the river are 30° and 45°, respectively. If the bridge is at a height of 3 m from the banks, find the width of the river.

Q15. An electrician has to repair an electric fault on a pole of height 5 m. He needs to reach a point 1.3 m below the top of the pole to undertake the repair work. What should be the length of the ladder that he should use which, when inclined at an angle of 60° to the horizontal, would enable him to reach the required position ? (Take $\displaystyle \sqrt{3}$ = 1.73)

Q16. A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree. Most Important

Q17. From the point P on the ground the angle of elevation of the top of a 10 m building is 30°. A flag is hoisted at the top of building and the angle of elevation of the top of flagstaff from P is 45º. Find the length of flagstaff and distance of building from P.

Q18. A 1.2m tall girl spots a balloon moving with the wind in a horizontal line at a height of 88.2 m from the ground. The angle of elevation of the balloon from the eyes of the girl at any instant is 60°. After some time, the angle of elevation reduces to 30°. Find the distance travelled by the balloon during the interval. Most Important

HBSE Class 10 Math Chapter 10 Circles Important Questions 2025

Q1. The lengths of tangents drawn from an external point to a circle are ___________.

Q2. A circle can have _______ parallel tangents at the most. Most Important

Q3. All circles are _________. (Similar/Congruent) Most Important

Q4. A tangent to a circle intersects it in __________ points.

Q5. A line intersecting a circle in two points is called ___________. Most Important

Q6. A tangent to a circle intersects it in _________ point(s). Most Important

Q7. The common point of a tangent to a circle and the circle is called __________. Most Important

Q8. From a point Q, the length of tangent to a circle is 24 cm and distance of Q from centre is 25 cm. Find the radius of circle.

Q9. Find the length of tangent drawn from a point whose distance from the centre of a circle is 25 cm. Given the radius of circle is 7 cm.

Q10. PQ is a chord of length 8 cm of a circle of radius 5 cm tangents at P and Q intersect at a point T. Find the length TP, if O is the centre of a circle.

Q11. Prove that the tangents drawn at the ends of a diameter of a circle are parallel.

Q12. Prove that the length of tangents drawn from an external point to a circle are equal. Most Most Important

Q13. Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that PTQ = 2 OPQ.

Q14. Prove that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.

Q15. Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Q16. Prove that the tangents drawn at the ends of a chord of a circle make equal angles with the chord.

HBSE Class 10 Math Chapter 11 Areas Related to Circles Important Questions 2025

Q1. Find the area of the sector of a circle with radius 7 cm and the angle at the centre is 30°. Most Most Important

Q2. A chord of a circle of radius 10 cm subtends an angle of 60° at the centre. Find the area of the corresponding sector.

Q3. The circumference of semi-circular piece of design is 72 cm. Find its area.

Q4. In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the length of arc.

Q5. Circumference of a circle is 22 cm, find the radius of the circle.

HBSE Class 10 Math Chapter 12 Surface Areas and Volumes Important Questions 2025

Q1. From a solid cylinder whose height is 2.4cm and diameter 1.4cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm².

Q2. Two cubes each of volume 64 cm³ are joined end to end. Find the surface area of the resulting cuboid. Most Important

Q3. A vessel is in the form of a hollow hemisphere mounted by a hollow cylinder. The diameter of the hemisphere is 14 cm and total height of the vessel is 13 cm. Find the inner surface area of the vessel.

Q4. A toy is in the form of a cone mounted on a hemisphere of radius 3.5 cm. The total height of the toy is 15.5 cm. Find the total surface area of the toy.

Q5. A cubical block of side 7 cm is surmounted by a hemisphere. What is the greatest diameter the hemisphere can have ? Find the surface area of solid.

Q6. A medicine capsule is in the shape of a cylinder with two hemisphere struck to each of its ends. The length of entire capsule is 14 mm and diameters of the capsule is 5 mm. Find its surface area.

Q7. Write the formula for finding out the curved surface area of the cylinder.

Q8. An underground water tank is in the form of a cuboid of edges 48 m, 36 m and 28m. Find the volume of the tank.

Q9. The volume of the right circular cone of height 21 cm and radius of its base 5 cm, is, _________.

Q10. Find the volume of the cone of height 24 cm and radius of base 6 cm.

Q11. The volume of the sphere of radius 8 cm is _________.

HBSE Class 10 Math Chapter 13 Statistics Important Questions 2025

Q1. 100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows:

Number of letters	1-4	4-7	7-10	10-13	13-16	16-19
Number of surnames	66	30	40	16	4	4

Find the median numbers of letters in the surnames.

Q2. The median of the following data is 28.5. Find the values of x and y, if the total frequency is 60.

Class interval	0-10	10-20	20-30	30-40	40-50	50-60
Frequency	5	x	20	15	y	5

Q3. Consider the distribution of daily wages of 50 workers of a factory :

Daily Wages (in Rs.)	100-120	120-140	140-160	160-180	180-200
Number of workers	12	14	8	6	10

Find the mean daily wages of the workers of the factory by using an appropriate method.

Q4. The following distribution shows the daily pocket allowance of children of a locality: The mean pocket allowance is Rs. 18. Find the missing frequency f.

Daily pocket (in Rs.)	11-12	13-15	15-17	17-19	19-21	21-23	23-25
Number of chapter	7	6	9	13	f	5	4

Q5. The following data gives the information on the life-time (in hours) of 75 electrical instruments:

Life-time (in hours)	0-20	20-40	40-60	60-80	80-100	100-120
Frequency	10	15	12	21	8	9

Q6. The median of the following data is 525. Find the value of x and y, if the total frequency is 100:

Class-Interval

Frequency

0-100

100-200

200-300

300-400

400-500

500-600

600-700

700-800

800-900

900-1000

Q7. A survey regarding the heights (in cm) of 51 girls of class X of a school was conducted and the following data was :

Obtained height (in cm)	Less than 140	Less than 145	Less than 150	Less than 155	Less than 160	Less than 165
Number of girls	4	11	29	40	46	51

Find the median height.

Q8. The table below shows daily expenditure on food of 25 households in a locality :

Daily Expenditure (in Rs.)	100-150	150-200	200-250	250-300	300-350
No. of households.	4	5	12	2	2

Find the mean daily expenditure on food by suitable method.

Q9. The following distribution shows the daily pocket money of children of a school:

Daily Pocket Money (Rs.)	11-13	13-15	15-17	17-19	19-21	21-23	23-25
Number of Children	7	6	9	13	20	5	4

Find the average daily pocket money of children.

Q10. The following distribution gives the monthly consumption of consumers of a locality. Find the median of the distribution.

Monthly consumption (in units)	65-85	85-105	105-125	125-145	145-165	165-185
Number of consumers	4	8	13	20	14	4

Q11. The wickets taken by a bowler in 10 cricket matches are as follows:
3 5 2 1 2 0 5 1 2 4
Find the mode of the data.

HBSE Class 10 Math Chapter 14 Probability Important Questions 2025

Q1. A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears (i) a perfect square number (ii) a number divisible by 5.

Q2. A lot of 20 bulbs contain 4 defective ones. One bulb is drawn at random from the lot. What is the probability that (i) this bulb is defective ? (ii) this bulb is not defective ?

Q3. A die is thrown once. Find the probability of getting (i) a prime number (ii) a number lying between 2 and 6 (iii) an odd number. Most Important

Q4. One card is drawn from a well-shuffled deck of 52 cards. Find the probability of getting (i) a king of red colour (ii) a face card. Most Most Important

Q5. A bag contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is (i) red (ii) not red? Most Most Important

Q6. A die is thrown once, find the probability of getting a prime number.

Q7. The probability of an event that cannot happen is __________ such an event called _______.

Q8. The probability of an event is greater than or equal to _________ and less than or equal to ________.

Q9. A die is thrown once. The probability of getting an odd number is __________.

Q10. If P(E) = 0.25, what is the probability of event ‘not E’.

Q11. Two dice are thrown at the same time. Find the probability of getting the sum on the dice is 13. Most Important

Q12. Two dice are thrown at the same time. Find the probability of getting the sum on the dice is less than or equal to 12.

Q13. If P(E) = 0.03, what is value of P (not E)?

HBSE Class 10 Math Important Question Answer 2025

HBSE Class 10 Math Chapter 1 Real Number Important Questions 2025

HBSE Class 10 Math Chapter 2 Polynomials Important Questions 2025

HBSE Class 10 Math Chapter 3 Pair of Linear Equations in Two Variables Important Questions 2025

HBSE Class 10 Math Chapter 4 Quadratic Equations Important Questions 2025

HBSE Class 10 Math Chapter 5 Arithmetic Progression Important Questions 2025

HBSE Class 10 Math Chapter 6 Triangles Important Questions 2025

HBSE Class 10 Math Chapter 7 Coordinate Geometry Important Questions 2025

HBSE Class 10 Math Chapter 8 Introduction to Trigonometry Important Questions 2025

HBSE Class 10 Math Chapter 9 Some Application of Trigonometry Important Questions 2025

HBSE Class 10 Math Chapter 10 Circles Important Questions 2025

HBSE Class 10 Math Chapter 11 Areas Related to Circles Important Questions 2025

HBSE Class 10 Math Chapter 12 Surface Areas and Volumes Important Questions 2025

HBSE Class 10 Math Chapter 13 Statistics Important Questions 2025

HBSE Class 10 Math Chapter 14 Probability Important Questions 2025

Share with Friends:

Related

Leave a Comment Cancel reply