HBSE Class 9 Math Important Question Answer 2025 PDF

NCERT Solution of Class 9 mathematics Important Question Answer solution with pdf. Here We Provides Class 1 to 12 all Subjects NCERT Solution with Notes, Question Answer, CBSE and HBSE Important Questions, MCQ and old Question Papers for Students.

HBSE ( Haryana Board ) Solution of Class 9 Math important Question And Answer solution of all chapters.

HBSE Class 9 Math Important Question Answer for 2025

HBSE Class 9 Math Chapter 1 Number System Important Questions 2025

Q1. The \displaystyle \frac{p}{q} form of \displaystyle 0.\overline{{47}} is _______ .

Q2. Rationalise the denominator of \displaystyle \frac{1}{{7+3\sqrt{2}}} .

Q3.  Rationalize the denominator of \displaystyle \frac{5}{{\sqrt{3}-\sqrt{5}}}

Q4. What will be get after rationalize the denominator of \displaystyle \frac{1}{{\sqrt{5}+\sqrt{2}}} ?

Q5. The value of \displaystyle (3+\sqrt{3})(2+\sqrt{2}) is

Q6. Find one rational number between 3 and 4.

Q7. Every rational number is an integer.  (True/False)

Q8. Simplify:  \displaystyle (3+\sqrt{3})(3-\sqrt{3})

Q9. Simplify : \displaystyle {{2}^{{\frac{2}{3}}}}{{.2}^{{\frac{1}{5}}}}

Q10. Express 0.3333…….= \displaystyle 0.\overline{3} in the form \displaystyle \frac{p}{q}, where p and q are integers and q ≠ 0.

Q11. Express 0.99999 _______ in the form \displaystyle \frac{p}{q}

Q12. Express \displaystyle 0.\overline{6} in the form \displaystyle \frac{p}{q} , where p and q are integers and q≠ 0.

HBSE Class 9 Math Chapter 2 Polynomials Important Questions 2025

Q1. Factorize the quadratic polynomial 6x2 + 5x – 6 .

Q2. Factorize : 27x3 + y3 + z3 – 9xyz Most Important

Q3. Factorise : 64m3 – 343m3 

Q4. Factorise : \displaystyle \frac{{{{x}^{2}}}}{{100}}-{{y}^{2}}

Q5. Factorise: 8a3 + b3 + 12a2b + 6ab2 Most Important

Q6.  Evaluate 103 x 107 without direct multiplication.

Q7. Factorize: 4x2 + 9y2 + 16z2 + 12xy – 24yz – 16xz

Q8. Factorise : x3 + 13x2 + 32x + 20

Q9. Factorise: x3 – 2x2 – x + 2

Q10. Evaluate 103 x 107 by using identity.

Q11. Without actually calculating the cubes, find the value of (28)3 + (−15)3 + (−13)3.

Q12. Using suitable identity, find the value of (102)2.

Q13. Find the value of k for which x – 1 is a factor of the polynomial x2 + x + k .  

Q14. Zero of p(x) = 3x + 1 is :

Q15. The coefficient of x2 in 2 – x2 +x3 is _________ .

Q16. The factors of 64m3 – 343n3 are __________.

Q17. What is product of ( x + 4 ) (x + 10)  ?

Ans – x2 + 14x + 40

Q18. Write \displaystyle {{[x-\frac{2}{3}y]}^{{-3}}}  in expanded form.

k का मान ज्ञात कीजिए, यदि x = 2, y = 1 समीकरण 2x + 3y = k का एक हल हैं  ।

Find the value of k, if x = 2, y = 1 is a solution of the equation 2x + 3y = k

k का मान ज्ञात कीजिए यदि x – 1, P(x) का गुणनखण्ड है P(x) = 2x2 + kx + √2

Find the value of k, if x – 1 is a factor of P(x), P(x) = 2x2 + kx + √2

गुणनखण्ड प्रमेय से x2 – 5x + 6 का गुणनखण्डन कीजिए।

Use the factor theorem factorize of x2 – 5x + 6 .

गुणनखण्ड कीजिए: 2x2 + 7x + 3

Factorize: 2x2 + 7x + 3

गुणनखण्ड कीजिए: 64x3 – 343y3

Factorize: 64x3 – 343y3

गुणनखण्ड कीजिए : 8x3 + y3 + 27z3 – 18xyz
Factorize: 8x3 + y3 + 27z3 – 18xyz

HBSE Class 9 Math Chapter 3 Coordinate Geometry Important Questions 2025

Q1. In which quadrant or on which axis do each of the points (-2, 4), (3, -1), (-1, 0), (1, 2) lie? Verify your answer by locating them on the Cartesian plane.

Q2. Plot the following ordered pairs (x, y) of numbers as points in the Cartesian Plane:

x -2 -3 3 0
y -3 7 -1 -1.5

Q3. Plot the following ordered pairs of numbers as points on the Cartesian Plane :

x 3 -2 -1 1
y -1 6 -4 -3

Q4. What is the name of each part of the plane formed by horizontal and the vertical lines in the Cartesian plane?

Q5. Point (5, -7) lies in which Quadrant ?

Q6. The point (0, 0) where x-axis and y-axis intersect is called _________ .

Q7. The abscissa and coordinate of (6, −4 ) is ________.

Q8. From the fig given below :

Write the answer of each of following from figure:

(i) The coordinates of B

(ii) The point identified by the coordinates (-3, -5)

(iii) The abscissa of the point D

(iv) The coordinate of point H

Q9.

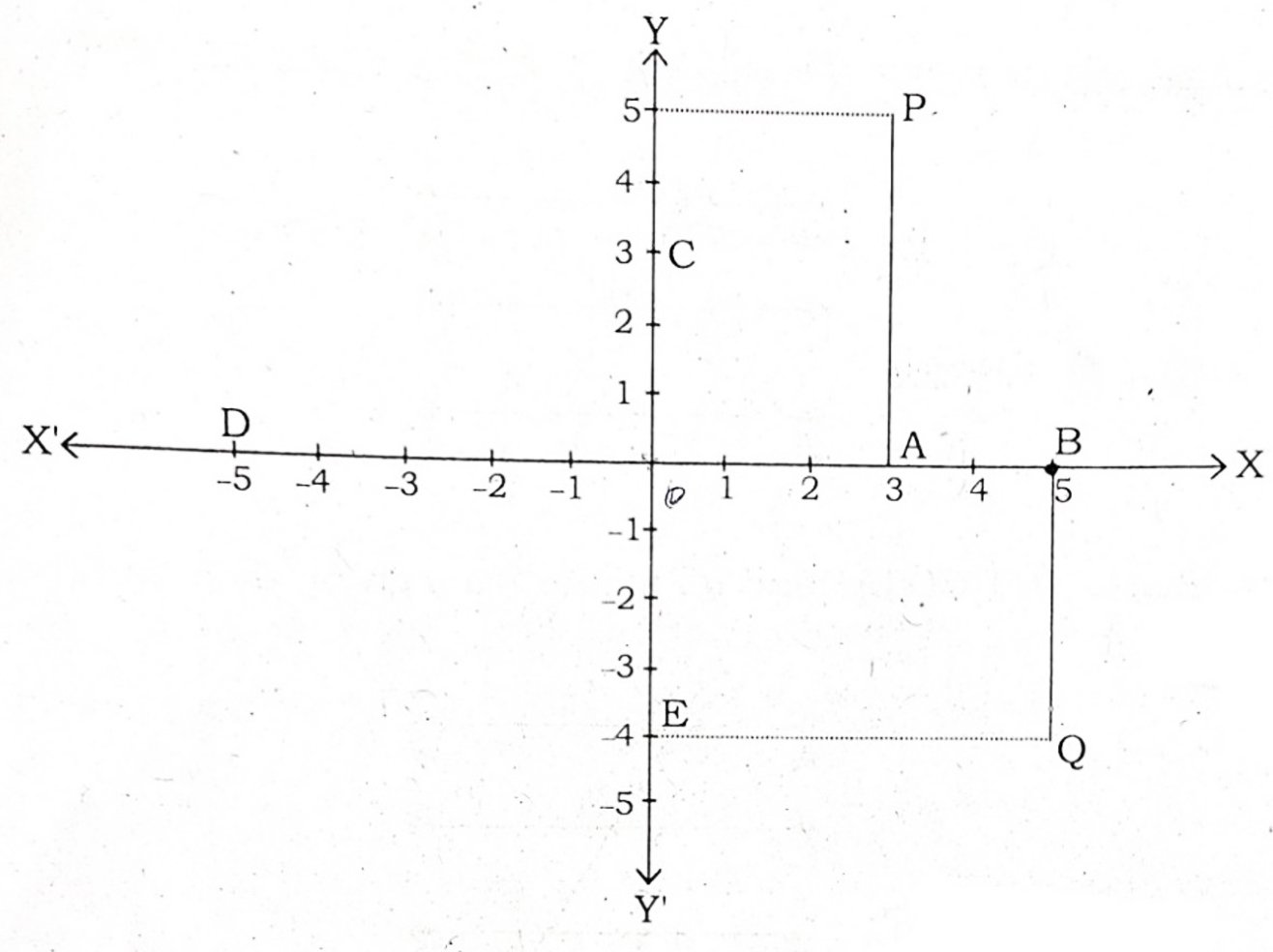
Look at the above figure and answer the following:
(i) Coordinates of A, B, C, D, E

(ii) Abscissa of point D

(iii) Ordinate of point E

(iv) Coordinates of point P, Q

HBSE Class 9 Math Chapter 4 Linear Equations in Two Variables Important Questions 2025

Q1. Find the four solutions for the equation 2x + y = 7 .

Q2. The floor of a rectangular hall has the perimeter 250 m. If the cost of the painting the four walls at the rate ₹ 12 per m2 is ₹ 18,000, find the height of the hall.

Q3. What is solution of (2x + 1) = x + 3

Q4. Solve the equation 2x + 1 = x – 3 and represent the solution on (i) The number line, (ii) The Cartesian plane.

Q5. Solve the equation 2x + 8 = 0 and represent the solution on (i) the number line (ii) the Cartesian plane.

Q6. Find four different solutions of the equation x+2y = 6.

Q7. Find the value of P if x = 2 , y = 1 is a solution of the equation 2x + 3y = P

Q8. The solution of a linear equation is not affected when the same number is added or subtracted from both the sides of the equation. (True/False)

Ans – True

HBSE Class 9 Math Chapter 5 Introduction to Euclid’s Geometry Important Questions 2025

Q1. What is second postulate of Euclid ?

Q2. Write the first postulate of Euclid.

Ans – A straight line may be drawn from any one point to any other point.

HBSE Class 9 Math Chapter 6 Lines and Angles Important Questions 2025

Q1. Write the name of the point where these two lines intersect.

Q2. Give a definition for each of the following terms:
(i) Parallel lines.
(ii) Line segment

Q3. Define supplementary angle. Give its two examples also.

Q4. In the given figure, AB || CD || EF and y: z = 3 : 7 are given, then find the value of x.

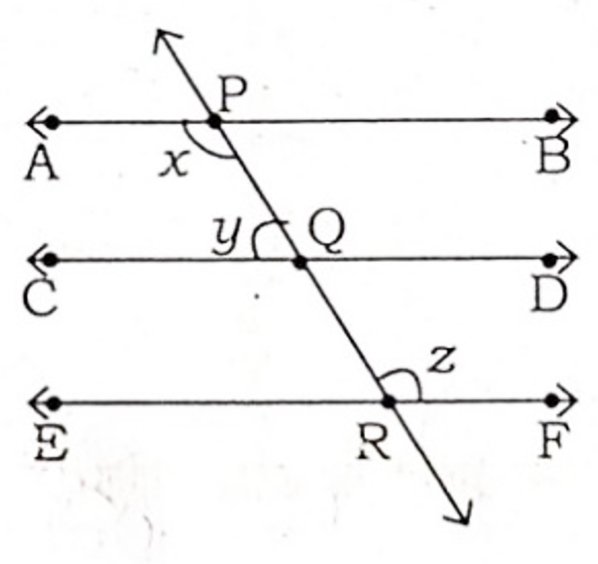
Q5. In fig., AB || CD and CD || EF. And EA ⊥ AB. If ∠BEF 55° then find the values of x, y and z.

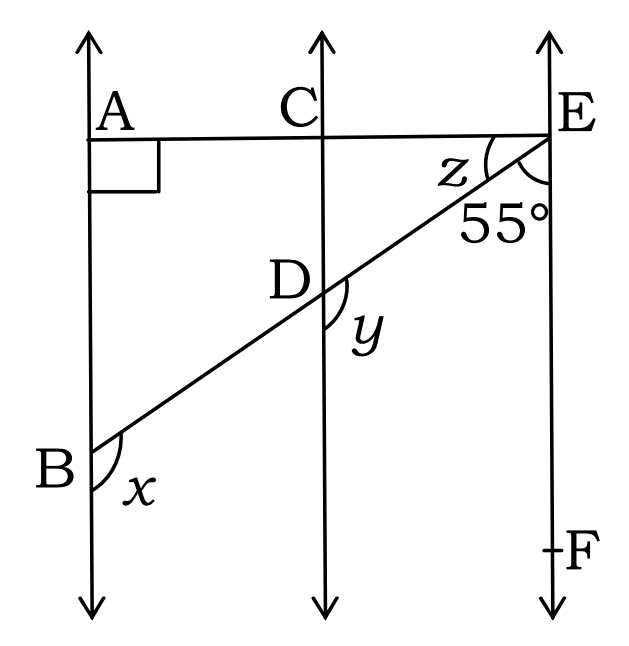
Q6. In fig., if PQ ⊥ PS, PQ || SR, ∠SQR = 28° and ∠QRT = 65°. Then find the values of x and y.

Q7. If in figure AC = BD, then prove that AB = CD : Most Important

Q8. A terminated line can be produced indefinitely. (True / False )

HBSE Class 9 Math Chapter 7 Triangles Important Questions 2025

Q1. Prove that angles opposite to equal sides of an isosceles triangle are equal.

Q2. Show that the angles of an equilateral triangle are 60° each. Most Important

Q3. Construct a triangle ABC in which BC = 8cm, ∠B = 45° and AB – AC = 3.5 cm. Most Important

Q4. ABC is a triangle right angled at C. A line through the mid point M of hypotenuse AB and parallel to BC intersects AC at D. Show that:

(i) D is the mid point of AC

(ii) MDIAC

(iii) CM = MA = \displaystyle \frac{1}{2}AB

Q5. Construct a triangle ABC in which BC = 7 cm, ∠B – 75° and AB + AC = 13 cm. Most Important

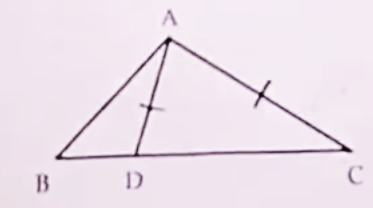
Q6. D is a point on side BC of ΔABC such that AD = AC in given figure show that AB > AD.

Q7. ΔABC is a right angled triangle in which ∠A = 90° and AB = AC. What is the value of ∠B and ∠C?

Q8. If ΔPRQ ≅ ΔCBA , then find:

(i) QR =…,.

(ii) PR =……

(iii) PO = …..

(iv) ∠B =…….

Q9. The triangle formed by joining the mid-points of the sides of an isosceles triangle is ________.

Q10. Construct a triangle ABC in which ∠B = 60°, ∠C = 45° and perimeter is 10 cm.   

Q11. To construct a triangle we must know at least its ________ parts .

Q12. The sum of any two sides of a triangle is _______ than the third side.

HBSE Class 9 Math Chapter 8 Quadrilaterals Important Questions 2025

Q1. If the diagonals of a parallelogram are equal, then show that it is a rectangle. 

Q2. Show that the diagonals of a rhombus are perpendicular to each other. Most Important

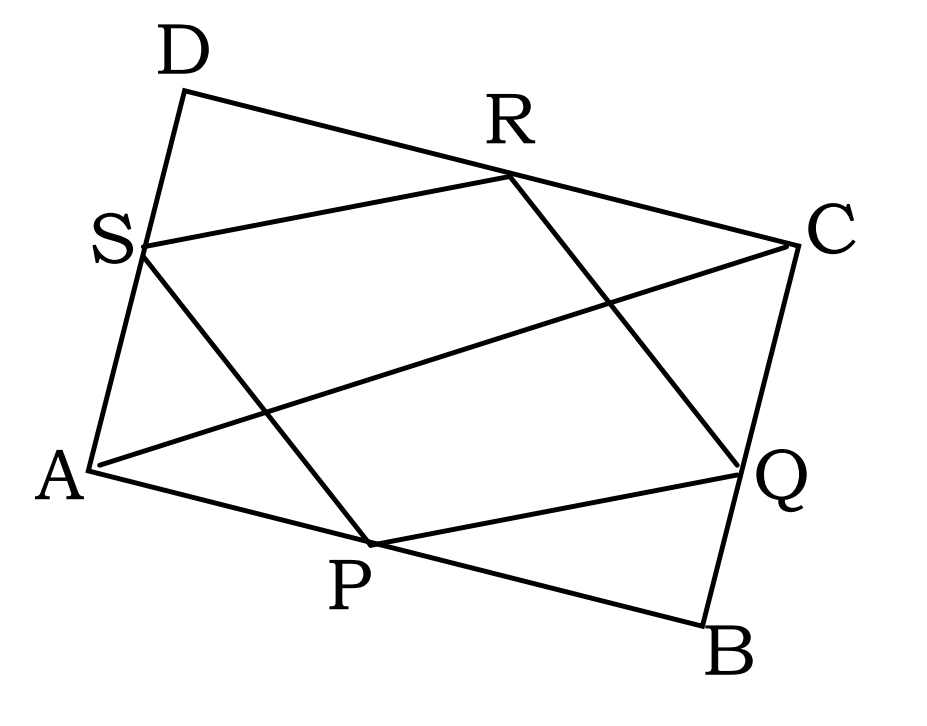
Q3. ABCD is a quadrilateral in which P, Q, R and S are mid points of the sides AB, BC, CD and DA. AC is a diagonal, show that:

(i) SR || AC and SR= \displaystyle \frac{1}{2} AC

(ii) PQ = SR

(iii) PORS is a parallelogram

Q4. If two sides of a cyclic quadrilateral are parallel, prove that:

(i) remaining two sides are equal and

(ii) both diagonals are equal

Q5. Show that the line segments joining the mid-points of opposite sides of a quadrilateral bisect each other.

Q6. In cyclic quadrilateral ABCD, AOC is the diameter of circle. Ir ∠CAD = 50°, then ∠ACD is :

Q7. The diagonals of a rectangle are _______ .

यदि एक चतुर्भुज के विकर्ण एक-दूसरे को समद्विभाजित करें, तो वह एक समान्तर चतुर्भुज होता है। (सत्य/असत्य)
If diagonal of a quadrilateral bisect each other, then quadrilateral is parallelogram. (True/False

HBSE Class 9 Math Chapter 9 Circles Important Questions 2025

Q1. Prove that a cyclic parallelogram is a rectangle.

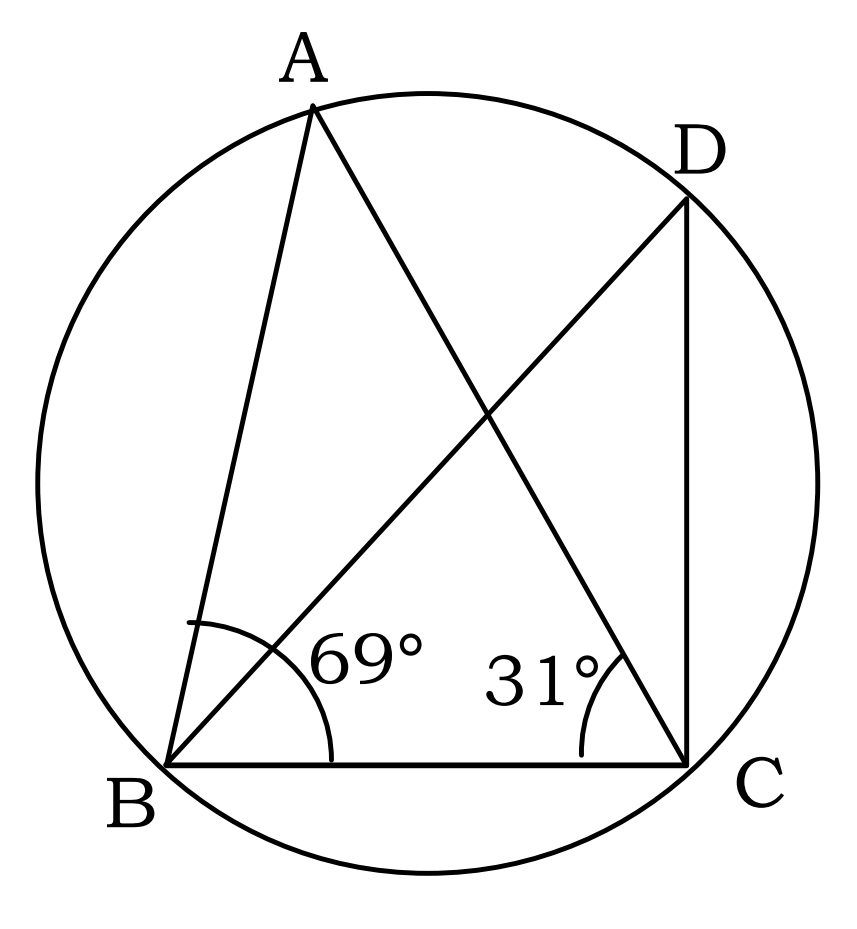
Q2. In figure angle ∠ABC = 69° and angle ∠ACB =31° find ∠BDC. Most important

Q3. A Roller 120 cm long has a diameter 84 cm. To level a playground, it takes 500 complete revolutions. Determine the area of playground in m2 .

Q4. Angles in the same segment of a circle are ______ .

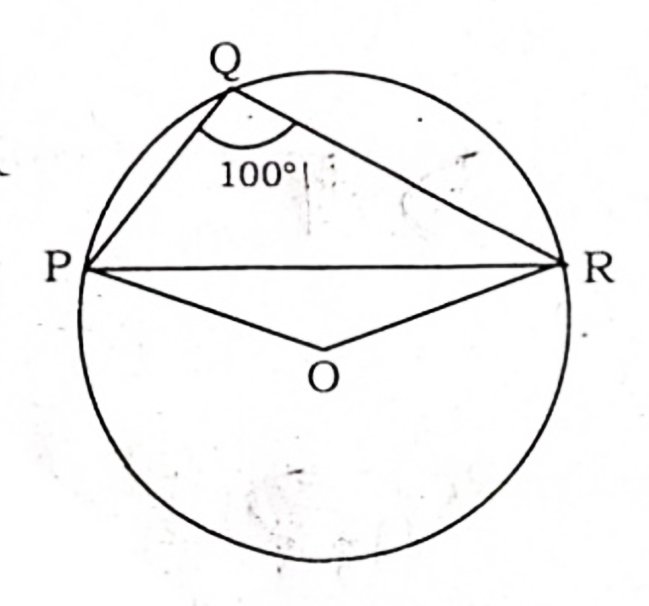
Q5. In the given figure ∠PQR = 100° , where P, Q and R are points on a circle with centre O. Find  ∠OPR .

Q6. In fig., A, B and C are three points on a circle with centre O such that ∠BOC = 30° and ∠AOB = 60°. If D is a point on the circle other than the are ABC, then ∠ADC will be:

Q7. A circle has only finite numbers of equal chords. (True/False)

Ans – False

HBSE Class 9 Math Chapter 10 Heron’s Formula Important Questions 2025

Q1. Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.

Q2. Sides of a triangle are in the ratio of 3 : 5 : 7 and its perimeter is 300 cm. Find its area.

HBSE Class 9 Math Chapter 11 Surface Areas and Volumes Important Questions 2025

Q1. Find the volume of right circular cone with radius 6 cm and height 7 cm. Most Important

Q2. The height of a cone is 15 cm. If its volume is 1570 cm3. the radius of it base is ?

Q3. The volume of a right circular cone is 9856 cm3. If the diameter of the base is 28 cm. Find :
(i) Height of the cone
(ii) Slant height of the cone
(iii) Curved surface area of the cone

Q4. A conical tent is 10 m high and the radius of its base is 24 m. Find:

(i) Slant height of the tent.

(ii) Cost of the canvas required to make the tent, if the cost of 1 m2 canvas is ₹ 70.

Q5. The inner diameter of a circular well is 3.5 m. It is 10 m deep. Find:

(i) Its inner curved surface area.

(ii) The cost of plastering this curved surface at the rate of Rs. 40 per m2.

Q6. Volume of a hemisphere with radius r will be ________ .

Q7. Find the total surface area of a hemisphere if its radius is 21 cm

Q8. Find the radius of a sphere whose surface area is 154 cm2.

HBSE Class 9 Math Chapter 12 Statistics Important Questions 2025

Q1. The following table gives the lifetimes of 400 neon lamps :

Lifetimes ( In Hours ) Number of Lamps
300-400

400-500

500-600

600-700

700-800

800-900

900-1000

 14

56

60

86

74

62

48

(i) Represent the given information with the help of a histogram.

(ii) How many lamps have a lifetime of more than 700 hours?

Q2. The Blood Groups of 30 students of class IX are recorded as follows:

A, B, O, O, AB, O, A, O, B, A, O, B, A, O, O, A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O

Represent this data in the form of a frequency distribution table.

Q3. A family with a monthly income of 6,400 plans his budget for a month as given below :

Grocery Clothing Education Miscellaneous Savings
2100 600 1200 1500 1000

 

(i) Represent the above data by a bar-graph.

(ii) On which item they spent more?

Q4. Class mark of class 140-150 is _________.

 

 

 

 

एक वृत्त (या सर्वांगसम वृत्तों) की समान जीवाएँ केन्द्र पर समान कोण बनाती हैं। (सत्य/असत्य)
Equal chords of a circle (or of congruent circles) subtend equal angles at the centre. (True/False

1 और 2 के बीच पाँच परिमेय संख्या ज्ञात कीजिए।
Find five rational numbers between 1 and 2.

सरल कीजिए: (3 + √3)(3 – √3)

Simplify: (3 + √3)(3 – √3)

अथवा
OR
सरल कीजिए : (32)1/5
Simplify: (32)1/5

 

103 x 107 का मान ज्ञात कीजिए।
Evaluate 103 x 107

 

अथवा
OR

 

खण्ड – ग

SECTION – C

 

एक अर्धगोलीय कटोरे की त्रिज्या 3 सेमी है इसमें पानी की मात्रा कितनी होगी ?

A hemispherical bowl has a radius of 3 it would contain.

OR

एक शंकु का कुल पृष्ठीय क्षेत्रफल ज्ञात कीजिए, यदि त्रिर्यक ऊँचाई 21 मीटर और इसके आधार का 24 मीटर है।

Find the total surface area of a cone, if its slant height is 21 m and diamet its base is 24 m.

 

समीकरण 2x + y = 7 के तीन भिन्न हल ज्ञात कीजिए।

Find three different solutions of the equation 2x + y = 7

 

 

अथवा
OR

उपयुक्त सर्वसमिका का प्रयोग करके (999)3 का मान ज्ञात कीजिए।
Using suitable identity, find the value of (999)3

SECTION – D

आकृति में रेखाएँ PQ और RS परस्पर बिन्दु पर प्रतिच्छेद करती हैं यदि ∠POR : ∠ROQ = 5 : 7 है, तो सभी कोण ज्ञात कीजिए।

In fig. PQ and RS line are intersect at point O. If ∠POR : ∠ROQ = 5 : 7 , then find the all angles.

33. यदि एक बिन्दु C दो बिन्दुओं A और B के बीच इस प्रकार स्थित है कि AC = BC है, तो सिद्ध कीजिए AC = 1/2AB चित्र बनाकर समझाइए।

If a point C lies between two points A and B such that AC = BC then prove that AC = 1/2AB Explain by drawing the figure.

अथवा
OR

चित्र में रेखाएँ XY और MN, O पर प्रतिच्छेद करती हैं। यदि ∠POY = 90° और a : b = 2 : 3 , तो c का मान ज्ञात कीजिए।

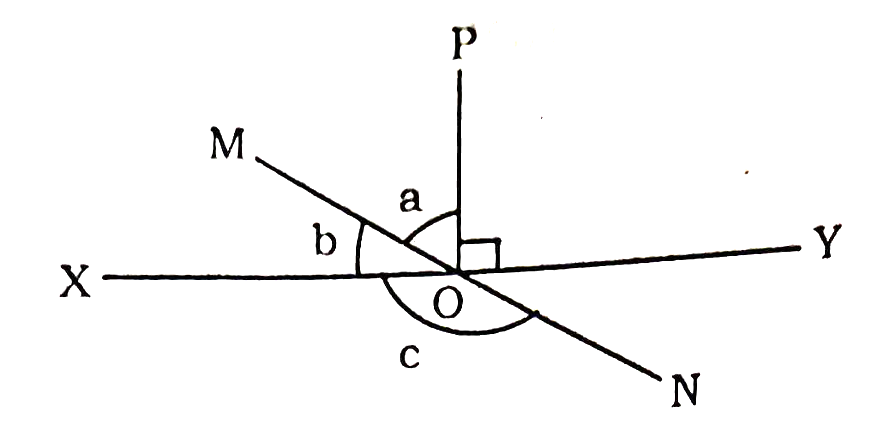
In fig. lines XY and MN intersect at O. If ∠POY = 90° and a : b = 2 : 3, find c.

34. एक त्रिभुज का क्षेत्रफल ज्ञात कीजिए जिसकी भुजाएँ 40 मी, 24 मी और 32 मी हैं।
Find the area of triangle whose sides are 40 m, 24 m and 32 m.

 

 

खण्ड – ङ
SECTION – E

36. आकृति देखकर निम्नलिखित प्रश्नों के उत्तर दीजिए :
(i) B के निर्देशांक।
(ii) निर्देशांक (-3,-5) द्वारा पहचाना गया बिन्दु।
(iii) (a) बिन्दु D का भुज तथा बिन्दु H की कोटि ज्ञात कीजिए।

(b) आकृति में रेखाखण्ड BD का X-अक्ष के साथ बनने वाले आयत का क्षेत्रफल ज्ञात कीजिए।

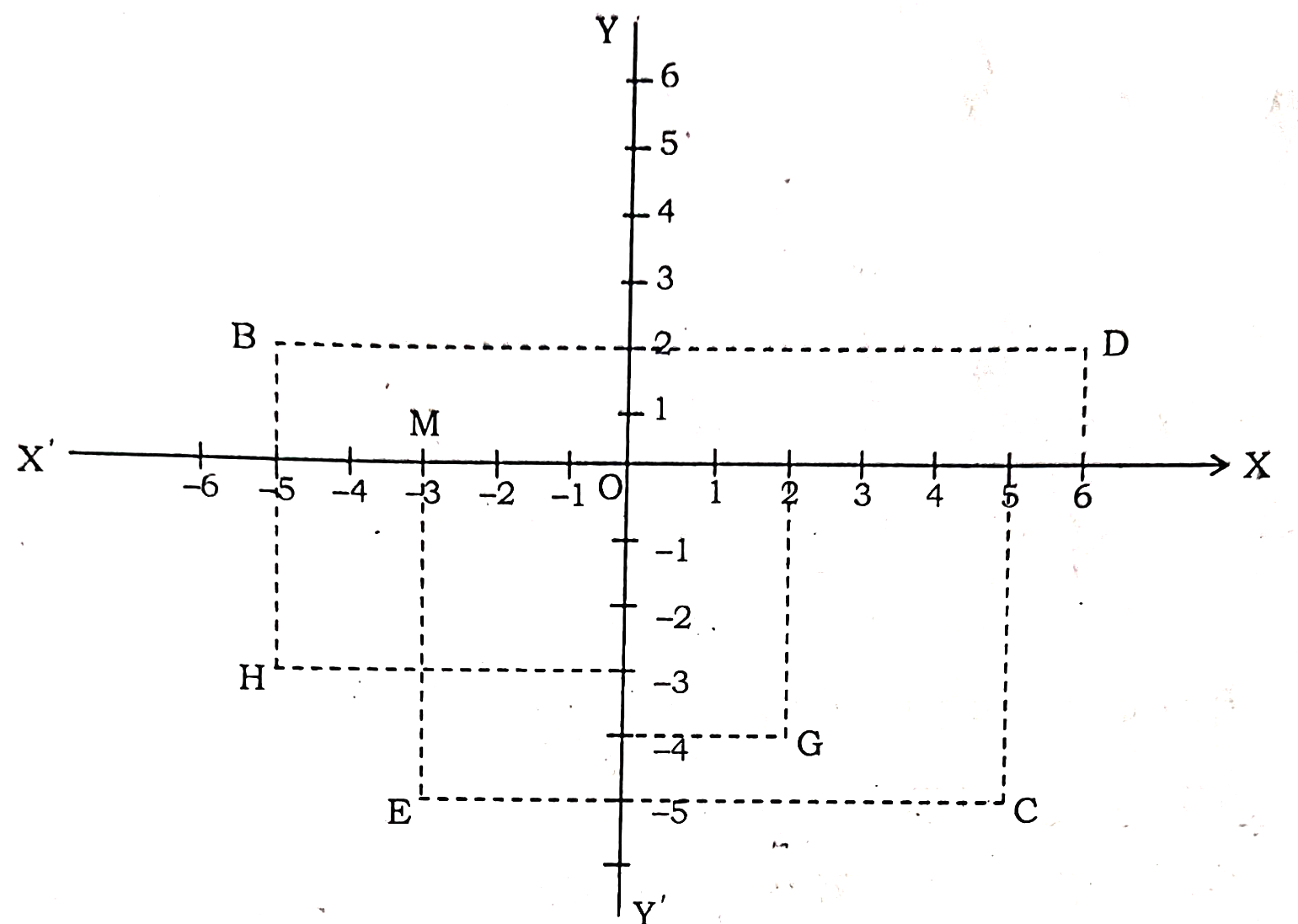
See the fig. and answer the following questions:
(i) The co-ordinate of B.
(ii) The point identified by the co-ordinate (-3, -5).
iii) (a) Find the abscissa of point D and ordinate of point H. (
OR
(b) Find the area of rectangle formed by the line segment BD and X-axis in fig.

37. विज्ञापन के लिए फ्लाईओवर की त्रिकोणीय साइड की दीवारों का उपयोग किया गया है। दीवारों की भुजाओं 122 मीटर, 22 मीटर और 120 मीटर हैं। विज्ञापनों से प्रति वर्ष 5000 रुपये प्रति मी2 की कमाई होनी है।
उपरोक्त जानकारी और दी गई आकृति के आधार पर निम्न प्रश्नों के उत्तर दीजिए:
(i) दीवार का परिमाप ज्ञात कीजिए।
(ii) हीरोन का सूत्र लिखिए।
(iii) (a) त्रिभुजाकार दीवार का क्षे० ज्ञात कीजिए।
अथवा
(b) यदि कम्पनी 1680 वर्ग मीटर क्षेत्रफल वाली एक दीवार को 3 महीने के लिए किराए पर लेती है, तो उसे कितना किराया देना होगा ?

The triangular side walls of the flyover have been used for advertising. The sides of the walls are 122 m, 22 m and 120 m. Earning of Rs. 5000 per m² per year from advertisements is.
On the basis of the above information and given figure, answer the following questions:
(i) Perimeter of wall is ____________.

(ii) Write down the Heron’s formula.
(iii) (a) Area of triangular wall is 1320 m²
OR
(b) If company hired one of its walls with area 1680 m² for 3 month, then how much rent did it pay ?

38. कक्षा IX के एक विशेष खण्ड में, 40 छात्रों से उनके जन्म महीनों के बारे में पूछा गया था और प्राप्त आँकड़ों के लिए निम्नलिखित ग्राफ तैयार किया गया था:
दिए गए दंड आलेख को देखें और निम्नलिखित प्रश्नों के उत्तर दीजिए :


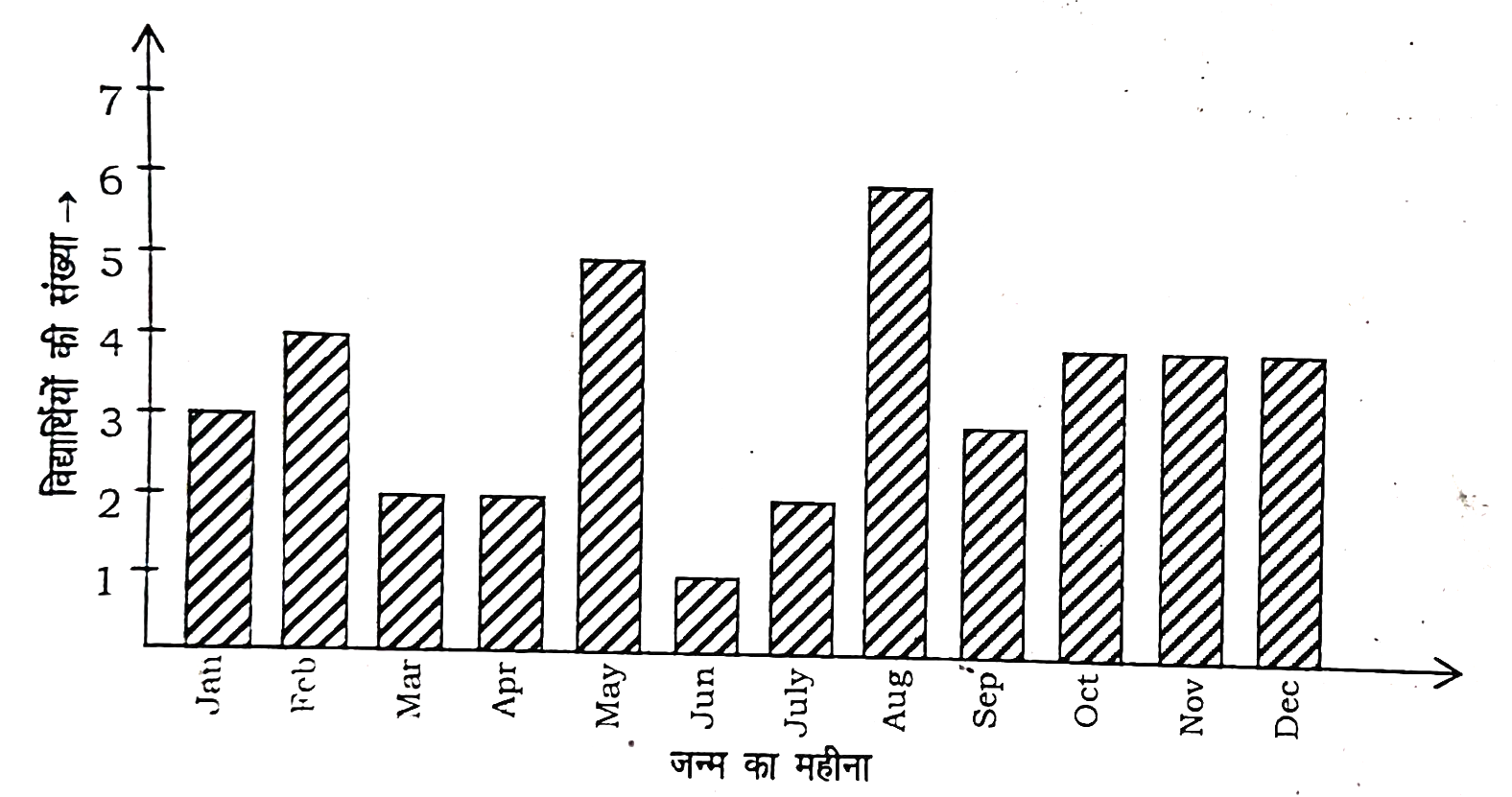
(i) नवंबर के महीने में कितने विद्यार्थियों का जन्म हुआ ?
(ii) किस महीने में सबसे अधिक विद्यार्थियों का जन्म हुआ ?
(iii)
(a) उन महीनों के नाम जिनमें 4 विद्यार्थियों का जन्म हुआ ?
अथवा
(b) मई से अगस्त के बीच पैदा हुए छात्रों की कुल संख्या ज्ञात कीजिए।

In a Particular section of IX Class, 40 students were asked about the months of their birth and the following graph was prepared for the data so obtained:
Observe the bar graph given below and answer the following questions:

(i) How many students were born in the month of November?
(ii) In which month were the maximum number of students born?
(iii) (a) Name the month in which 4 students were born.
OR
(b) Find the total number of students born from May to August.

 

 

Leave a Comment