NCERT Class 10 Math Chapter 13 Important Questions Answer – Statistics

Class 10
Chapter  Statistics
Subject Math
Category Important Question Answer

Class 10 Math Chapter 13 Important Question Answer

Q1. 100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows:

Number of letters 1-4 4-7 7-10 10-13 13-16 16-19
Number of surnames  6 30 40 16 4 4

Find the median numbers of letters in the surnames.

Ans.

Number of letters Number of surnames Cumulative Frequency
1-4

 

4-7

 

7-10

 

10-13

 

13-16

 

16-19

 6

 

30

 

40

 

16

 

4

 

4

 6

 

36

 

76

 

92

 

96

 

100

 

Now, n=100, \displaystyle \frac{n}{2}=\frac{{100}}{2}=50. This observation lies in the class 7-10.

Then, l (lower limit) = 7,

cf (the cumulative frequency of the class preceding 7-10) = 36

f (the frequency of the median class 7-10) = 40,

h (the class size) = 3.

Using the formula, Median = l + \displaystyle \left( {\frac{{\frac{n}{2}-cf}}{f}} \right) × h

Median =7 + \displaystyle \left( {\frac{{50-36}}{{40}}} \right)\times 3 =7  + 1.05 = 9.05

Q2. The median of the following data is 28.5. Find the values of x and y, if the total frequency is 60.

Class interval 0-10 10-20 20-30 30-40 40-50 50-60
Frequency 5 x 20 15 y 5

 

Ans.

Class-Interval Frequency Cumulative Frequency
0-10

 

10-20

 

20-30

 

30-40

 

40-50

 

50-60

 

 5

 

x

 

20

 

15

 

y

 

5

 5

 

5 + x

 

25 + x

 

40 + x

 

40 + x + y

 

45 + x + y

It is given that n = 60

So, 45 + x + y = 60  i.e., x + y = 15

The Median is 28.5, which lies in the class 20-30

So, l = 20,  f = 20,  cf = 5 + x,  h = 10

Using the formula, Median = l + \displaystyle \left( {\frac{{\frac{n}{2}-cf}}{f}} \right) × h

28.5 = 20 + \displaystyle \left( {\frac{{30-5-x}}{{20}}} \right)\times 10

28.5  – 20 = \displaystyle \frac{{25-x}}{2}

17 = 25 – x

x = 8

putting vlaue of x in x + y = 15,

we get y = 7

Q3. Consider the distribution of daily wages of 50 workers of a factory :

Daily Wages (in Rs.) 100-120  120-140 140-160 160-180 180-200
Number of workers 12 14 8 6 10

Find the mean daily wages of the workers of the factory by using an appropriate method.

Ans.

As the given data is large, we will use step deviation method to find the mean.

Here a = 150, h = 20

Class Interval Frequency

(fi)

 Class Marks

(ui)

 \displaystyle {{u}_{i}}=\frac{{{{x}_{i}}-a}}{h} fiui
100-120

120-140

140-160

160-180

180-200

 12

14

8

6

10

 110

130

150 (a)

170

190

 -2

-1

0

1

2

 

 -24

-14

0

6

20
\displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}}}=50 \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}}}{{u}_{i}}=-12

 

Step Deviation Method 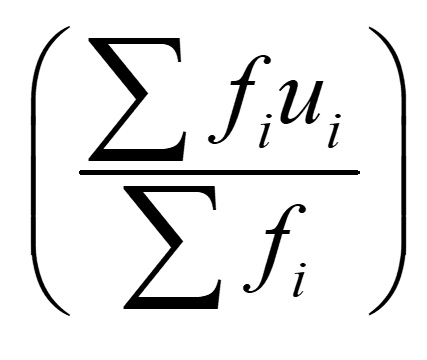: \displaystyle \overline{x} = a + × h

= 150 + \displaystyle 20\left( {\frac{{-12}}{{50}}} \right) = 150 – \displaystyle \frac{{240}}{{50}}

= 145.20

Hence, the mean daily expenditure on food is Rs.145.20

Q4. The following distribution shows the daily pocket allowance of children of a locality: The mean pocket allowance is Rs. 18. Find the missing frequency f.

Daily pocket (in Rs.) 11-13 13-15 15-17 17-19 19-21 21-23 23-25
Number of chapter 7 6 9 13 f 5 4

Ans.

Daily pocket allowances ( in Rs.) Class Mark

(xi)

 Number of children

(fi)

 Di = xi – 18 fidi
11-13

13-15

15-17

17-19

19-21

21-23

23-25

 12

14

16

18 ( let a = 18)

20

22

24

 7

6

9

13

f

5

4

 -6

-4

-2

0

2

4

6

 -42

-24

-18

0

2f

20

24
Total \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}=44+f}} \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}{{d}_{i}}=2f-40}}

We have,

Assumed Mean Method : \displaystyle \overline{x} = a +

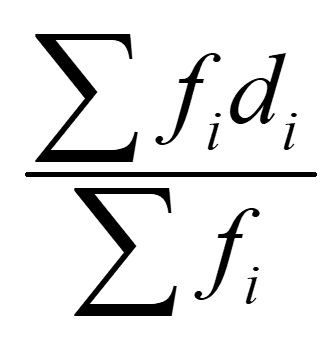
18 = 18 + \displaystyle \frac{{2f-40}}{{44+f}}

0 = \displaystyle \frac{{2f-40}}{{44+f}}

2f – 40 = 0

f = 20

Therefore, required frequency is 20.

Q5. The following data gives the information on the life-time (in hours) of 75 electrical instruments:

Life-time (in hours) 0-20 20-40 40-60 60-80 80-100 100-120
Frequency 10 15 12 21 8 9

Determine the modal lifetime of the components.

Ans.

Here the maximum frequency is 21. So the modal class is 60-80.

Now,

Modal class = 60-80, lower limit (l) of modal class = 60, class size (h) = 20

Frequency (f1) of modal class = 21

Frequency (f0) of class preceding the modal class = 12,

Frequency (f2) of class succeeding the modal class = 8

Now, Using Mode = l + 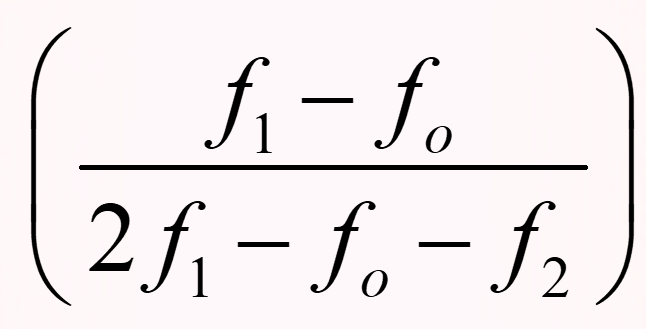× h

Mode = 60 + \displaystyle \left( {\frac{{21-12}}{{2(21)-12-8}}} \right)\times 20 = 60 + \displaystyle \frac{{90}}{{11}}= 68.18

Q6. The median of the following data is 525. Find the value of x and y, if the total frequency is 100:

Class-Interval Frequency
0-100

100-200

200-300

300-400

400-500

500-600

600-700

700-800

800-900

900-1000

 2

5

x

12

17

20

y

9

7

4

Ans.

Class-Interval Frequency Cumulative Frequency
0-100

 

100-200

 

200-300

 

300-400

 

400-500

 

500-600

 

600-700

 

700-800

 

800-900

 

900-1000

 2

 

5

 

x

 

12

 

17

 

20

 

y

 

9

 

7

 

4

 2

 

7

 

7+x

 

19 + x

 

36 + x

 

56 + x

 

56 + x + y

 

65 + x + y

 

72 + x + y

 

76 + x + y

 

 It is given that n = 100

So, 76 + x + y = 100  i.e., x + y = 24

The Median is 525, which lies in the class 500-600

So, l = 500,  f = 20,  cf = 36 + x,  h = 100

Using the formula, Median = l + \displaystyle \left( {\frac{{\frac{n}{2}-cf}}{f}} \right) × h

525 = 500 + \displaystyle \left( {\frac{{50-36-x}}{{20}}} \right)\times 100

525 – 500 = (14 – x)×5

25 = 70 – 5x

5x = 70 – 25 = 45

x = 9

putting value of x in x + y = 24,

we get 9 + y = 24

y = 15

Q7. A survey regarding the heights (in cm) of 51 girls of class X of a school was conducted and the following data was :

Obtained height (in cm) Less than 140 Less than 145 Less than 150 Less than 155 Less than 160 Less than 165
Number of girls 4 11 29 40 46 51

Find the median height.

Ans.

The given distribution being of the less than type. Therefore, the classes should be below 140, 140-145, 145-150, ….., 160-165.

The frequency of class interval below 140 is 4, frequency of class interval 140-145 is 11-4=7. Similarly the frequency of 145-150 is 29-11=18 and so on.

So, our frequency distribution table with the given cumulative frequencies becomes :

Class Interval Frequency Cumulative Frequency  (cf)
Below 140 4 4
140-145 7 11
145-150 18 29
150-155 11 40
155-160 6 46
160-165 5 51

Now, n=51, \displaystyle \frac{n}{2}=\frac{{51}}{2}=25.5. This observation lies in the class 145-150.

Then, l (lower limit) = 145,

cf (the cumulative frequency of the class preceding 145-150) = 11

f (the frequency of the median class 145-150) = 18,

h (the class size) = 5.

Using the formula, Median = l + \displaystyle \left( {\frac{{\frac{n}{2}-cf}}{f}} \right) × h

Median = 145 + \displaystyle \left( {\frac{{25.5-11}}{{18}}} \right)\times 5

= 145 + \displaystyle \frac{{72.5}}{{18}} = 149.03

Q8. The table below shows daily expenditure on food of 25 households in a locality :

Daily Expenditure (in Rs.) 100-150 150-200 200-250 250-300 300-350
No. of households. 4 5 12 2 2

Find the mean daily expenditure on food by suitable method.

Ans.

Here, a = 225 and h = 50

Class Interval Frequency Class Marks \displaystyle {{u}_{i}}=\frac{{{{x}_{i}}-a}}{h} fiui
100-150

150-200

200-250

250-300

300-350

 4

5

12

2

2

 125

175

225 (a)

275

325

 -2

-1

0

1

2

 

 -8

-5

0

2

4
\displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}}}=25 \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}}}{{u}_{i}}=-7

Step Deviation Method 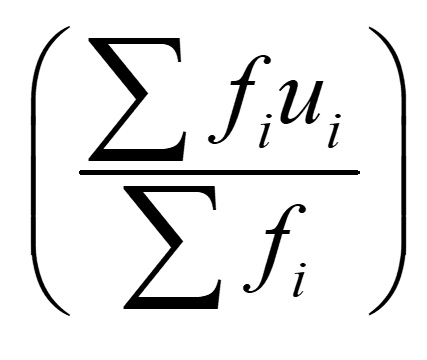: \displaystyle \overline{x} = a + × h

= 225 + \displaystyle 50\left( {\frac{{-7}}{{25}}} \right) = 225 – 14

= 211.

Hence, the mean daily expenditure on food is Rs.211.

Q9. The following distribution shows the daily pocket money of children of a school:

Daily Pocket Money (Rs.) 11-13  13-15  15-17 17-19  19-21 21-23 23-25
Number of Children 7 6 9 13 20 5 4

 Find the average daily pocket money of children.

Ans.

Daily pocket allowances ( in Rs.) Class Mark

(xi)

 Number of children

(fi)

 Di = xi – 18 fidi
11-13

13-15

15-17

17-19

19-21

21-23

23-25

 12

14

16

18 ( let a = 18)

20

22

24

 7

6

9

13

20

5

4

 -6

-4

-2

0

2

4

6

 -42

-24

-18

0

40

20

24
Total \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}=64}} \displaystyle \sum\limits_{{}}^{{}}{{{{f}_{i}}{{d}_{i}}=0}}

 

We have,

Assumed Mean Method : \displaystyle \overline{x} = a +

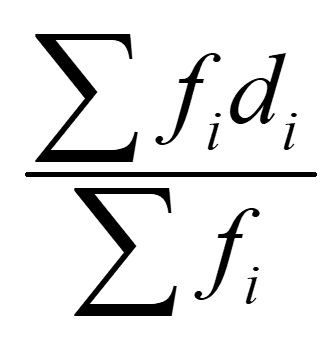
\displaystyle \overline{x} = 18 + \displaystyle \frac{0}{{64}} = 18

Therefore, the average daily pocket money of children is Rs. 18.

Q10. The following distribution gives the monthly consumption of consumers of a locality. Find the median of the distribution.

Monthly consumption (in units) 65-85 85-105 105-125 125-145 145-165 165-185
Number of consumers 4 8 13 20 14 4

Ans.

Monthly consumption

 (in units)

 Number of consumers

(f)

 Cumulative Frequency

 (cf)
65-85 4 4
85-105 8 12
105-125 13 25
125-145 20 45
145-165 14 59
165-185 4 63

Now, n = 63, \displaystyle \frac{n}{2}=\frac{{63}}{2}=31.5. This observation lies in the class 125-145 .

Then, l (lower limit) = 125,

cf (the cumulative frequency of the class preceding 125-145) = 25,

f (the frequency of the median class 125-145) = 20,

h (the class size) = 20.

Using the Formula, Median = l + \displaystyle \left( {\frac{{\frac{n}{2}-cf}}{f}} \right) × h

Median = 125 + \displaystyle \left( {\frac{{31.5-25}}{{20}}} \right)20 = 125 + 6.5 = 126.5

Q11. The wickets taken by a bowler in 10 cricket matches are as follows:
3    5     2    1     2     0    5     1     2     4
Find the mode of the data.

Ans. Arranging data in ascending order : 0, 1, 2, 2, 2, 3, 4, 5, 5

From above data we can clearly conclude that 3 is the mode of data as it is used in max times.

Therefore,

Mode = 3

Also Read Class 10 Math NCERT Solution
Also Read Class 10 Important Questions [Latest]

Leave a Comment

error: